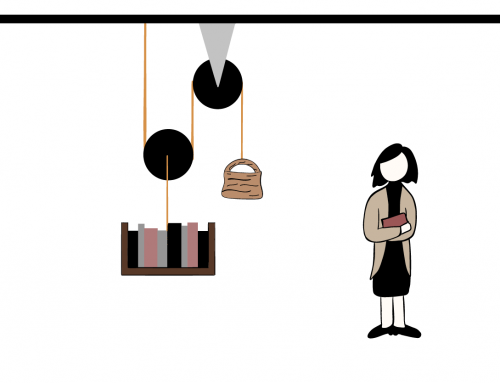
A commemorative trophy is collecting dust on a shelf where it was placed years ago after a historic win. Time has taken its toll, and the shelf’s hanging mechanism has begun to deteriorate. If the left edge of the shelf suddenly breaks free, as shown in the figure above, write an expression in terms of \(\mu\) and \(g\) (the gravitational constant) for the maximum angle \(\theta\) that the shelf can form with the floor before the trophy starts sliding. Your answer may contain the variable, \(\mu\) which represents the coefficient of static friction between the shelf and the trophy’s base.
Draw a free-body diagram; it may be easier if you rotate the shelf to coincide with the \({x-}\)axis. Be careful with the angle for the weight because it will have components along both the \({x-}\) and \({y-}\)axes. Find the force due to friction and the normal force, and you should be able to solve it relatively easily.
The maximum force due to static friction is:
\begin{equation*}
fr_{max} = \mu N.
\end{equation*}
Newton’s Second Law along the \({x-}\)axis yields:
\begin{equation*}
mg \sin \theta – fr_{max}=0,
\end{equation*}
and Newton’s Second Law along the \({y-}\)axis yields:
\begin{equation*}
N-mg\cos \theta=0.
\end{equation*}
Solving for \(\mu\) in the first equation, and using the expressions for \(fr_{max}\) and \(N\) from the second and third equation respectively, we get:
\begin{equation*}
\tan \theta = \mu,
\end{equation*}
which gives
\begin{equation*}
\theta = \arctan ( \mu ).
\end{equation*}
For a more detailed explanation of any of these steps, click on “Detailed Solution”.
To find the maximum angle \(\theta\) that the shelf can form with the floor before the trophy starts sliding, we need to relate that angle to other known variables such as \(\mu\) and \(g\). To find the relationship between these variables, it is helpful to start with a free body diagram representing all known forces. First, let’s choose a coordinate system. As is common with inclined planes, we will use a coordinate where the \({x-}\)axis points along the direction of motion, and \({y}\) is perpendicular to the surface (see Figure 1).
Figure 1: We choose the coordinate system with the same inclination as the broken shelf with the X axis pointing down the inclined shelf and the Y axis perpendicular to it.
There are three forces acting on the trophy: the weight (which is vertical and downwards), the normal force (which is perpendicular to the surface), and the force due to static friction (which opposes the trophy’s tendency to move due to the weight.) In this case, the trophy tends to slide along the positive \({x-}\)axis due to the \({x-}\)component of the weight, and so the force due to static friction points in the direction of the negative \({x-}\)axis, as shown in Figure 2.
Figure 2: Force diagram for the cup with the following forces shown: the contact force with the shelf \(N\), the friction \(f_r\), and the weight \(W\).
In general, there is no single equation for the force due to static friction, since the force due to friction can vary a lot. For example, if the angle of the shelf is very small, the trophy will not slide very much. Therefore, little friction is needed to counterbalance the trophy’s tendency to slide; on the other hand, as the angle increases, the stronger the \({x-}\)component of the weight, which corresponds to a greater potential to slide. In that case, to prevent the trophy from sliding, the coefficient of static friction must be high. In this problem, we want to find the maximum angle before the trophy slides. We must therefore consider the maximum possible static friction. This friction is given by
\begin{equation}
\label{Trophy_friccion}
fr_{max} = \mu N,
\end{equation}
where \(\mu\) is the coefficient of static friction, and \(N\) the magnitude of the normal force. From this, we get
\begin{equation}
\label{Trophy_mu}
\frac{fr_{max}}{N} = \mu
\end{equation}
To find the coefficient of static friction, we need to find \(fr_{max}\) and \(N\). For \(fr_{max}\), we can use the equations along \({x}\). According to the free body diagram, there are two forces in the \({x-}\)direction: the force due to static friction (which is negative) and the \({x-}\)component of the weight which is positive:
\begin{equation}
W_x \, \hat{\textbf{i}} – fr_{max} \, \hat{\textbf{i}} = m a_x \, \hat{\textbf{i}}.
\end{equation}
Now, an important condition in the prompt is that the trophy does not slide. This means we can set \(a_x\) to 0:
\begin{equation}
W_x \, \hat{\textbf{i}} – fr_{max} \, \hat{\textbf{i}} = 0 \, \hat{\textbf{i}}.
\end{equation}
Furthermore, we can use, from the diagram, that \(W_x=W \sin \theta\). If we use this and focus on the magnitudes, we get
\begin{equation}
\label{Trophy_friccionParaReemplazar}
W \sin \theta = fr_{max},
\end{equation}
The last thing we need to find in order to use equation \eqref{Trophy_mu} is \(N\). To do so, consider again the free body diagram. There are only two forces along \({y}\): the \({y-}\)component of the weight (which is negative) and the normal force (which is positive). Hence,
\begin{equation}
N \, \hat{\textbf{j}} – W_y \, \hat{\textbf{j}} = m a_y \, \hat{\textbf{j}}.
\end{equation}
But there is no acceleration in the \({y-}\)direction, and so
\begin{equation}
N \, \hat{\textbf{j}} – W_y \, \hat{\textbf{j}} = 0 \, \hat{\textbf{j}}.
\end{equation}
From the free body diagram, we see that \(W_y= W\cos \theta\). If we utilize this relationship and focus on the magnitudes, we get
\begin{equation}
N – W \cos \theta = 0,
\end{equation}
written more plainly as
\begin{equation}
N = W \cos \theta.
\end{equation}
Finally, we can use this result together with \eqref{Trophy_friccionParaReemplazar} in equation \eqref{Trophy_mu}:
\begin{equation}
\frac{W \sin \theta}{W \cos \theta} = \mu,
\end{equation}
which is
\begin{equation}
\tan \theta = \mu.
\end{equation}
Or explicitly,
\begin{equation}
\theta = \arctan ( \mu ).
\end{equation}
This angle is known as the critical angle (it is critical because any angle greater than this one will make the object slide). Notice that it does not depend on the mass of the object!
You need to be registered and logged in to take this quiz. Log in



Leave A Comment